Skip to content
Technical University of Munich

Events

AdONE Seminar: Prof. Serpil Sayın (Koç University)

Prof. Prof. Serpil Sayın (Koç University): Representations of the Nondominated Set in Multiobjective Optimization – The solution to a multiobjective optimization problem consists of the nondominated set that portrays all relevant trade-off information. This set is often difficult to obtain and present to a Decision Maker. Therefore it makes sense to try to identify a Decision Maker’s most preferred solution without generating the entire nondominated set; however, Decision Makers may not always be able to express their true preferences without knowing the entire trade-off landscape. Thus the representation idea suggests finding a representative subset of the nondominated set that conforms to some prespecified quality measure.

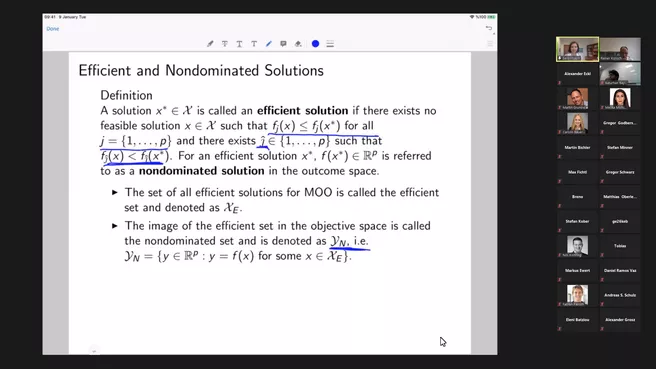
In this talk, we provide a quick overview of representation approaches for multiobjective optimization.  We then focus on a bilevel programming subproblem that is capable of delivering a nondominated solution that comes from a  given set, provided that one exits. If the Decision Maker’s preferences are known a priori, they can be used to specify the given set. Alternatively, we describe how the subproblem can be used  to obtain a representation of the nondominated set when the Decision Maker’s preferences are not available. This requires a thorough search of the outcome space. The search can be facilitated by a partitioning scheme similar to the ones used in global optimization. While building a discrete representation, the algorithm also generates an approximation of the nondominated set within the specified coverage error. We illustrate the algorithm on the multiobjective linear programming problem.  We discuss potential application areas and possibilities for further research.

◄ Back to: Events
To top